Orbital mechanics

Max and min orbital speed

Posted on by John

An earlier post needed to calculate how much the speed of a planet varies in orbit. The planet moves fastest as perihelion, the point in its orbit closes to the sun, and it moves slowest at aphelion, when it is furthest from the sun.

The ratio of the maximum to minimum speed turns out to be a simple expression in terms of the eccentricity e of the orbit:

(1 + e)/(1 − e).

You can derive this fairly quickly from the vis-viva equation, which in turn is derived from conservation of energy.

There are several things I find interesting about this. First, that the expression is so simple. Second, it can be simplified even more for small e:

(1 + e)/(1 − e) ≈ 1 + 2e.

This comes from expanding the ratio as a series:

(1 + e)/(1 − e) = 1 + 2e + 2e² + 2e³ …

This explains two things from the previous post. First, that the variation in orbital speed, for both Earth and Mars, worked out to be about 2e. The eccentricity of Earth’s orbit is 0.0167 and orbital speed varies by about 3%. Mars’ orbit has eccentricity 0.0934 and its orbital speed varies by about 19%. Since the eccentricity of Mars orbit, while fairly small, is larger than that of Earth, the quadratic term matters more for Mars.

Finally, “I keep running into the function f(z) = (1 − z)/(1 + z),” as I first wrote four years ago, and wrote on again a few months ago. It comes up, for example, in computing impedance, in mental calculation tricks, and in efficient calculation of the perimeter of an ellipse. Now you can add to that list calculating the variation in orbital speed in a two body problem.

A calendar for Mars

Posted on by John

I recently started reading The Case for Mars by Robert Zubrin. This post will unpack one line from that book regarding creating a calendar for Mars:

Equipartitioned months don’t work for Mars, because the planet’s orbit is elliptical, which causes the seasons to be of unequal length.

This sentence doesn’t sit well at first for a couple reasons. First, Earth’s orbit is elliptical too, and the seasons here are of roughly equal length. Second, the orbit of Mars, like the orbit of Earth, is nearly circular.

There are three reasons why Zubrin’s statement is correct, despite the objections above. The first has to do with the nature of eccentricity, and the second with the reference to which angles are measured, and the third with variable speed.

Eccentricity

The orbit of Mars is about five and a half times as eccentric as that of Earth. That does not mean that the orbit of Mars is noticeably different from a circle, but it does mean the sun is noticeably not at the center of that (almost) circle.

There’s a kind of paradox interpreting eccentricity e. An ellipse with e = 0 is a circle, and the two foci of the ellipse coincide with the center of the circle. As e increases, the ellipse aspect ratio increases and the foci move apart. But here’s the key: the aspect ratio doesn’t change nearly as fast as the distance between the two foci changes. I’ve written more about this here and here.

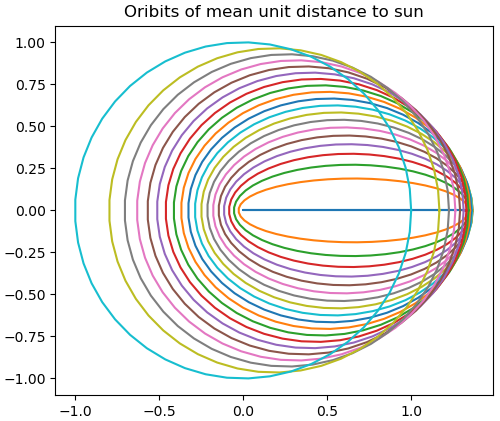
So while the orbit of Mars is nearly a circle, the sun is substantially far from the center of the orbit. We can visualize this with a couple plots. First, here are the orbits of Earth and Mars, shifted so that both have their center at the origin.

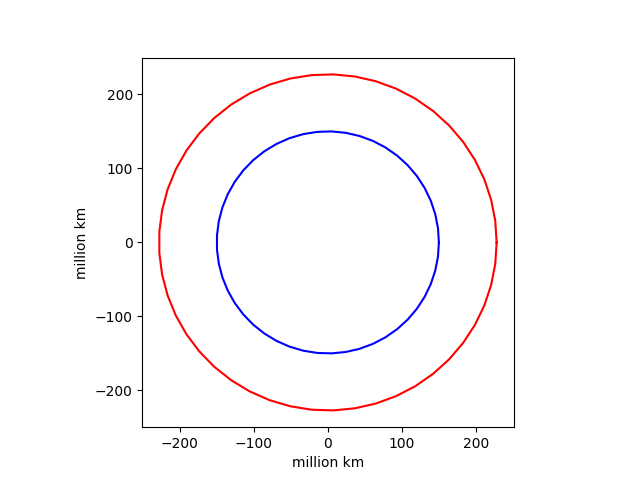
Both are visually indistinguishable from circles.

How here are the two orbits with their correct placement relative to the sun at the center.

Angle reference

Zubrin writes

In order to predict the seasons, a calendar must divite the planet’s orbit not into equal division of days , but into equal angles of travel  around the sun. … a month is really 30 degrees of travel around the Sun.

If we were to divide the orbit of Mars into partitions of 30 degrees relative to the center of the orbit then each month would be about the same length. But Zubrin is dividing the orbit into partitions of 30 degrees relative to the sun.

In the language of orbital mechanics, Zubrin’s months correspond to 30 degrees of true anomaly, not 30 degrees of mean anomaly. I explain the difference between true anomaly and mean anomaly here. That post shows that for Earth, true anomaly and mean anomaly never differ by more than 2 degrees. But for Mars, the two anomalies can differ by up to almost 19 degrees.

Variable speed

A planet in an elliptical orbit around the sun travels fastest when it is nearest the sun and slowest when it is furthest from the sun. Because Mars’s orbit is more eccentric than Earth’s, the variation in orbital speed is greater. We can calculate the ratio of the fastest speed to the slowest speed using the vis-viva equation. It works to be

(1 + e)/(1 − e).

For Earth, with eccentricity 0.0167 this ratio is about 1.03, i.e. orbital speed varies by about 3%.

For Mars, with eccentricity 0.0934 this ratio is about 1.21, i.e. orbital speed varies by about 21%.

Zubrin’s months

The time it takes for Mars to rotate on its axis is commonly called a sol, a Martian day. The Martian year is 669 sols. Zubrin’s proposed months range from 46 to 66 sols, each corresponding to 30 degrees difference in true anomaly.

Related posts

Starlink configurations

Posted on by John

My nephew recently told me about being on a camping trip and seeing a long line of lights in the sky. The lights turned out to be Starlink satellites. It’s fairly common for people report seeing lines of these satellites.

Four lights in the sky in a line

Why would the satellites be in a line? Wouldn’t it be much more efficient to spread them out? They do spread out, but they’re launched in groups. Satellites released into orbit at the same time initially orbit in a line close together.

It would seem the optimal strategy would be to spread communication satellites out evenly in a sphere. There are several reasons why that is neither desirable or possible. It is not desirable because human population is far from evenly distributed. It’s very nice to have some coverage over the least-populated places on earth, such as Antarctica, but there is far more demand for service over the middle latitudes.

It is not possible to evenly distribute more than 20 points on a sphere, and so it would not be possible to spread out thousands of satellites perfectly evenly. However there are ways to arbitrarily many points somewhat evenly, such as in a Fibonacci lattice.

It’s also not possible to distribute satellites in a static configuration. Unless a satellite is in geostationary orbit, it will constantly move relative to the earth. One problem with geostationary orbit is that it is at an altitude of 42,000 km. Starlink satellites are in low earth orbit (LEO) between 300 km and 600 km altitude. It is less expensive to put satellites into LEO and there is less latency bouncing signals off satellites closer to the ground.

Satellites orbit at different altitudes, and altitude and velocity are tightly linked. You want satellites orbiting at different altitudes to avoid collisions, they’re orbiting at different velocities. Even if you wanted all satellites to orbit at the same altitude, this would require constant maintenance due to various real-world departures from ideal Keplerian conditions. Satellites are going to move around relative to each other whether you want them to or not.

Related posts

The vis-viva equation

Posted on by John

The vis-viva equation greatly simplifies some calculations in orbital mechanics. It is reminiscent of how conservation of energy can sometimes trivialize what appears to be a complicated problem. In fact, the vis-viva equation is derived from conservation of energy, but the derivation is not trivial. Which is good: the effort required in the derivation implies the equation is a shortcut to a place that might take longer to arrive at starting from first principles.

The term vis viva is Latin for “life force” and was applied to mechanics by Liebnitz around 350 years ago. The vis-viva equation is also known as the vis-viva law or the vis-viva integral.

The vis-viva equation says that for an object orbiting another object in a Keplerian orbit

v^2 = \mu\left(\frac{2}{r} - \frac{1}{a}\right)

Here v is the relative velocity of the two bodies, r is the distance between their centers of mass, and a is the semi-major axis of the orbit. The constant μ is the standard gravitational parameter. It equals the product of the gravitational constant G and the combined mass M of the two bodies.

In practice it is often accurate enough to let M be the mass of the larger object; this works for GPS satellites circling the earth but would not be adequate to describe Charon orbiting Pluto.

For a circular orbit, r = a and so v² = μ/r. Then r is constant and v is constant.

More generally a is constant but r is continually changing, and the vis-viva equation says how r and v relate at any given instant. This shows, for example, that velocity is smallest when distance is greatest.

For an object to escape the orbit of another, the ellipse of its orbit has to become infinitely large, i.e. a → ∞. This says that if v is escape velocity, v² = 2μ/r. For a rocket on the surface of a planet, r is the radius of the planet. But for an object already in a high orbit, escape velocity is less because r is larger.

Related posts

GPS satellite orbits

Posted on by John

GPS satellites all orbit at the same altitude. According to the FAA,

GPS satellites fly in circular orbits at an altitude of 10,900 nautical miles (20,200 km) and with a period of 12 hours.

Why were these orbits chosen?

You can determine your position using satellites that are not in circular orbits, but with circular orbits all the satellites are on the surface of a sphere, and this insures that certain difficulties don’t occur [1]. More on that in the next post.

To maintain a circular orbit, the velocity is determined by the altitude, and this in turn determines the period. The period T is given by

T = 2\pi \sqrt{\frac{r^3}{\mu}}

where μ is the “standard gravitational parameter” for Earth, which equals the mass of the earth times the gravitational constant G.

The weakest link in calculating of T is r. The FAA site says the altitude is 20,200 km, but has that been rounded? Also, we need the distance to the center of the earth, not the altitude above the surface, so we need to add the radius of the earth. But the radius of the earth varies. Using the average radius of the earth I get T = 43,105 seconds.

Note that 12 hours is 43,200 seconds, so the period I calculated is 95 seconds short of 12 hours. Some of the difference is due to calculation inaccuracy, but most of it is real: the orbital period of GPS satellites is less than 12 hours. According to this source, the orbital period is almost precisely 11 hours 58 minutes.

The significance of 11 hours and 58 minutes is that it is half a sidereal day, not half a solar day. I wrote about the difference between a sidereal day and a solar day here. That means each GPS satellite returns to almost the same position twice a day, as seen from the perspective of an observer on the earth. GPS satellites are in a 2:1 resonance with the earth’s rotation.

(But doesn’t the earth rotate on its axis every 24 hours? No, every 23 hours 56 minutes. Those missing four minutes come from the fact that the earth has to rotate a bit more than one rotation on its axis to return to the same position relative to the sun. More on that here.)

Update: See the next post on the mathematics of GPS.

[1] Mireille Boutin, Gregor Kemperc. Global positioning: The uniqueness question and a new solution method. Advances in Applied Mathematics 160 (2024)

Increasing speed due to friction

Posted on by John

Orbital mechanics is fascinating. I’ve learned a bit about it for fun, not for profit. I seriously doubt Elon Musk will ever call asking me to design an orbit for him. [1]

One of the things that makes orbital mechanics interesting is that it can be counter-intuitive. For example, atmospheric friction can make a satellite move faster. How can this be? Doesn’t friction always slow things down?

Friction does reduce a satellite’s tangential velocity, causing it to move into a lower orbit, which increases its velocity. It’s weird to think about, but the details are worked out in [2].

Note the date on the article: May 1958. The paper was written in response to Sputnik 1 which launched in October 1957. Parkyn’s described the phenomenon of acceleration due to friction in general, and how it applied to Sputnik in particular.

Related posts

[1] I had a lead on a project with NASA once, but it wasn’t orbital mechanics, and the lead didn’t materialize.

[2] D. G. Parkyn. The Effect of Friction on Elliptic Orbits. The Mathematical Gazette. Vol. 42, No. 340 (May, 1958), pp. 96-98

Music of the spheres

Posted on by John

The idea of “music of the spheres” dates back to the Pythagoreans. They saw an analogy between orbital frequency ratios and musical frequency ratios.

HD 110067 is a star 105 light years away that has six known planets in orbital resonance. The orbital frequencies of the planets are related to each other by small integer ratios.

The planets, starting from the star, are labeled b, c, d, e, f, and g. In 9 “years”, from the perspective of g, the planets complete 54, 36, 24, 16, 12, and 9 orbits respectively. So the ratio of orbital frequencies between each pair of consecutive planets are either 3:2 or 4:3. In musical terms, these ratios are fifths and fourths.

In the chord below, the musical frequency ratios are the same as the orbital frequency ratios in the HD 110067 system.

Here’s what the chord sounds like on a piano:

hd11067.wav

Related posts

Set of orbits with the same average distance to sun

Posted on by John

Suppose a planet is in an elliptical orbit around the sun with semimajor axis a and  semiminor axis b. Then the average distance of the planet to the sun over time equals

a(1 + e²/2)

where the eccentricity e satisfies

e² = 1 − b²/a².

You can find a proof of this statement in [1].

This post will look at the set of all orbits with a fixed average distance r to the sun. Without loss of generality we can choose our units so that r = 1.

Clearly one possibility is to set a = b = 1 so the orbit is a circle. The distance is constantly 1, so the average is 1.

We can also maintain a distance of 1 by reducing a but increasing the eccentricity e. The possible orbits of average distance 1 satisfy

a(1 + e²/2) = 1

with 0 < b ≤ a ≤ 1. A little algebra shows that

b = √(3a² – 2a),

and that 2/3 < a ≤ 1. As a approaches 2/3, b approaches 0.

Let’s put the center of our coordinate system at the sun and assume the other focus of the elliptical orbits is somewhere along the positive x-axis. When e is 0 we have a unit circle orbit. As e approaches 1, the orbits approach a horizontal line with the sun on one end.

Related posts

[1] Sherman K. Stein. “Mean Distance” in Kepler’s Third Law. Mathematics Magazine, Vol. 50, No. 3 (May, 1977), pp. 160–162

Dutton’s Navigation and Piloting

Posted on by John

This morning Eric Berger posted a clip from The Hunt for Red October as a meme, and that made me think about the movie.

I watched Red October this evening, for the first time since around the time it came out in 1990, and was surprised by a detail in one of the scenes. I recognized one of the books: Dutton’s Navigation and Piloting.

Screen shot with Dutton's Navigation and Piloting

I have a copy of that book, the 14th edition. The spine looks exactly the same. The first printing was in 1985, and I have the second printing from 1989. So it is probably the same edition and maybe even the same printing as in the movie. I bought the book last year because it was recommended for something I was working on. Apparently it’s quite a classic since someone thought that adding a copy in the background would help make a realistic set for a submarine.

My copy has a gold sticker inside, indicating that the book came from Fred L. Woods Nautical Supplies, though I bought my copy used from Alibris.

Here’s a clip from the movie featuring Dutton’s.

Dutton’s has a long history. From the preface:

Since the first edition of Navigation and Nautical Astronomy (as it was then titled) was written by Commander Benjamin Dutton, U. S. Navy, and published in 1926, this book has been updated and revised. The title was changed after his death to more accurately reflect its focus …

The 14th edition contains a mixture of classical and electronic navigation, navigating by stars and by satellites. It does not mention GPS; that is included in the latest edition, the 15th edition published in 2003.

Related posts

Oval orbits?

Posted on by John

Johannes Kepler thought that planetary orbits were ellipses. Giovanni Cassini thought they were ovals. Kepler was right, but Cassini wasn’t far off.

In everyday speech, people use the words ellipse and oval interchangeably. But in mathematics these terms are distinct. There is one definition of an ellipse, and several definitions of an oval. To be precise, you have to say what kind of oval you have in mind, and in the context of this post by oval I will always mean a Cassini oval.

Ellipses and ovals each have two foci, f1 and f2. Let d1(p) and d2(p) be the distances from a point p to each of the foci. For an ellipse, the sum d1(p) + d2(p) is constant. For an oval, the product d1(p) d2(p) is constant.

In [1] the authors argue that just as planetary orbits are nearly circles, they’re also nearly ovals. This post will look at how far the earth’s orbit is from a circle and from an oval.

We need a way to specify which oval we want to compare to the ellipse of earth’s orbit. We’ll do this by equating the major and minor semi-axes of the two curves. These are usually denoted a and b, but the same variables have a different meaning in the context of ovals, so I’ll denote them by M for major and m for minor.

The equation of an ellipse is

(x/M)² + (y/m)² = 1

and the equation of an oval is

((x + a)² + y²) ((x – a)² + y²) = b².

Setting x = 0 in the equation of an oval tells us

m² = b – a²

and setting y = 0 tells us

M² = b + a².

So

b = (M² + m²)/2

and

a² = (M² – m²)/2.

For the earth’s orbit, M = 1.00000011 and m = 0.99986048 measured in AU, astronomical units. So or oval has parameters

a = 0.011816102

and

b = 0.99986060.

If you plot Kepler’s ellipse and Cassini’s oval for earth’s orbit at the same time, you can’t see the difference.

Planet orbits are nearly circular. If we compare a circle of radius 1 AU with Kepler’s ellipse we get a maximum error of about 1 part in 10,000.

But if we compare Cassini’s oval with Kepler’s ellipse we get a maximum error of about 1 part in 100,000,000.

 

In short, a circle is a good approximation to earth’s orbit, but a Cassini oval is four orders of magnitude better.

It would be difficult to empirically distinguish an ellipse from an oval as the shape of earth’s orbit, but theory is clearly on Kepler’s side since his ellipses fall out of Newton’s laws. Cassini’s error was more qualitative than quantitative.

More orbital mechanics posts

[1] Kepler’s ellipse, Cassini’s oval and the trajectory of planets. B Morgado1 and V Soares. 2014 Eur. J. Phys. 35 025009 DOI 10.1088/0143-0807/35/2/025009