The exponential sum of the day page draws a new figure each day by plotting the partial sums of
and drawing a line between consecutive terms. Here m, d, and y are the month, day, and last two digits of the year.
Today’s sum has an unusual number of lines that are parallel to the horizontal and vertical axes.
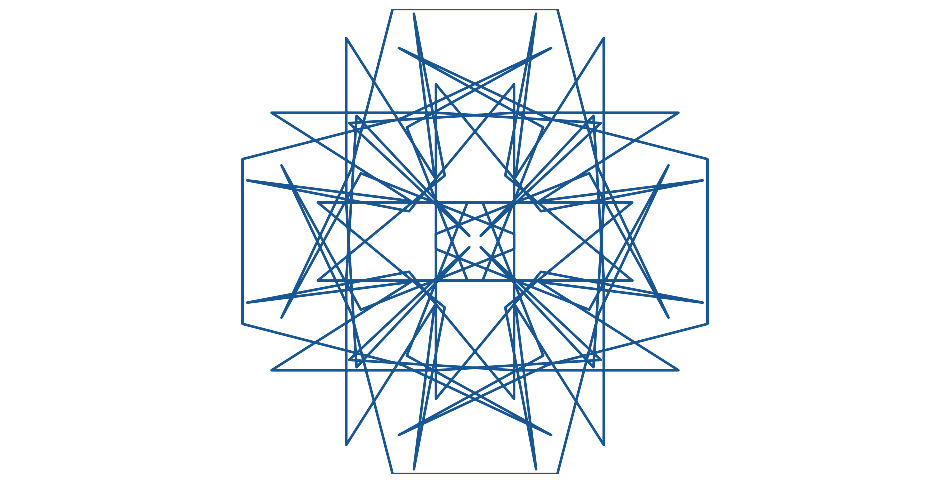
Typically the images on the exponential sum of the day page are much more complicated, except around the first of the month.
Horizontal lines occur when consecutive partial sums differ by a real number, i.e. when the latest term in the sum is real. Similarly, vertical lines occur when the latest term is purely imaginary. This happens when n is a multiple of 10.
Also, nearly horizontal or vertical lines occur when the latest term is nearly real or purely imaginary. This happens a few times as well.
