The Fermat curve of order n is the set of points satisfying
xn + yn = 1
for a positive integer n. Fermat’s last theorem is equivalent to saying there are no non-trivial rational points on the Fermat curve of order n > 2. (The trivial points have x or y equal to 0.)
Parameterization
The Fermat curve of order 2 is a circle, which can be parameterized by (cos t, sin t) for 0 ≤ t < 2π.
The Dixon elliptic functions cm and sm, mentioned in the previous post, satisfy
cmn x + smn y = 1
and so the Fermat curve of order 3 can be parameterized by (cm t, sm t). What is the necessary range on t?
Period
In the previous post we said that the function sm maps the unit circle to an equilateral triangle. One of the vertices of this triangle is
v = ⅓ B(⅓, ⅓)
in the complex plane, and the other two vertices are rotations of this vertex about the origin. We could have defined v by
v = π3 / 3
where
π3 = B(⅓, ⅓).
The Dixon functions are periodic with period π3 [1] and we can take for our parameterization −⅓ π3 < t < ⅔ π3.
Plot
Here’s a plot of the Fermat curve of order 3.
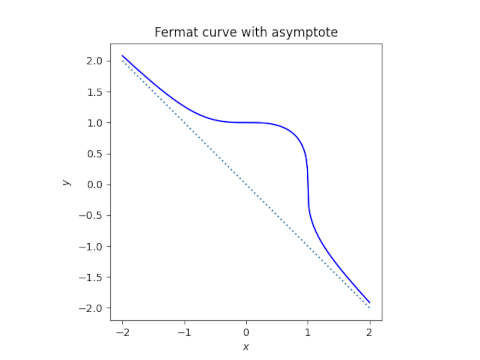
In the first quadrant, the Fermat curve is a squircle.
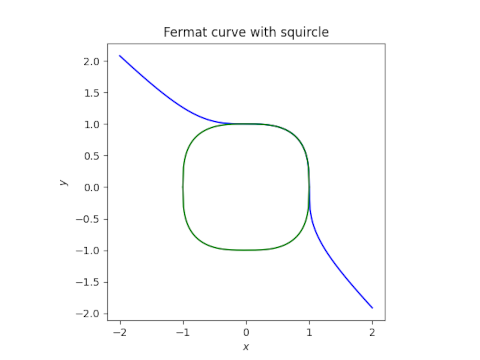
The equation of a squircle is the same as that of a Fermat curve, except you take the absolute value of the arguments. The exponent is usually denoted p rather than n and can be any real number p > 2 rather than a positive integer.
Related posts
[1] Because the Dixon functions are elliptic functions, they are periodic in two independent directions. The Dixon functions are also periodic in the direction exp(2πi/3), and the period is also of length π3 in that direction. Elliptic functions can have different period lengths in different directions, but for the Dixon functions the two period lengths are the same.
