Here are a dodecahedron (left) and icosahedron (right) made from Zometool pieces.
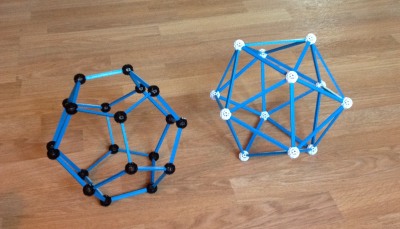
These figures are duals of each other: If you put a vertex in the middle of each face of one of the shapes, and connect all the new vertices, you get the other shape. You could use these as a tangible way to introduce duality to kids.
There are lots of patterns that kids might discover for themselves. The dodecahedron has 12 faces and 20 vertices; the icosahedron has 20 faces and 12 vertices. At each vertex of the dodecahedron 3 five-sided faces come together; at each vertex of the icosahedron 5 three-sided faces come together.
The two polyhedra have the same number of edges. You can see this by taking one shape apart to make the other. A more sophisticated explanation is that Euler’s theorem says that V + F = E + 2. When you swap the roles of V and F, V+F doesn’t change, so E cannot change.
Here’s a hint on making an icosahedron with Zometool. Stick the red struts with the pentagonal ends into every pentagonal hole on one of the balls. Now if you connect each of the outer balls to each other, you have an icosahedron. You can leave the red pieces inside, or you can use a few of them as a temporary scaffolding to get started, then remove them.
If you do leave the red pieces inside, it’s hard to put the last few pieces in place because the shape is so rigid.


In the special case of dual polyhedra, I submit that the Right Way to see that the number of edges is the same for both is to observe that they’re in 1-1 correspondence: the edge shared by faces a,b corresponds to the edge joining vertices A,B.
Beautiful!
More interesting is the fact that that is a d12 on the left, and a d20 on the right. The platonic solids are used for dice in games such as D&D.
Also the d10, which isn’t a platonic solid, but we use it anyway.